Suggested Pre-lab Assignment

1. Read Section 3.4 of the E-book and list 5 components of the rough vacuum system with short description of their functionality.

2. Read Section 3.5 of the E-book and draw a symbol for each vacuum system component from Question 1.

Theoretical Background

Figure 2.2 shows cylindrically-shaped object with diameter D and height H.

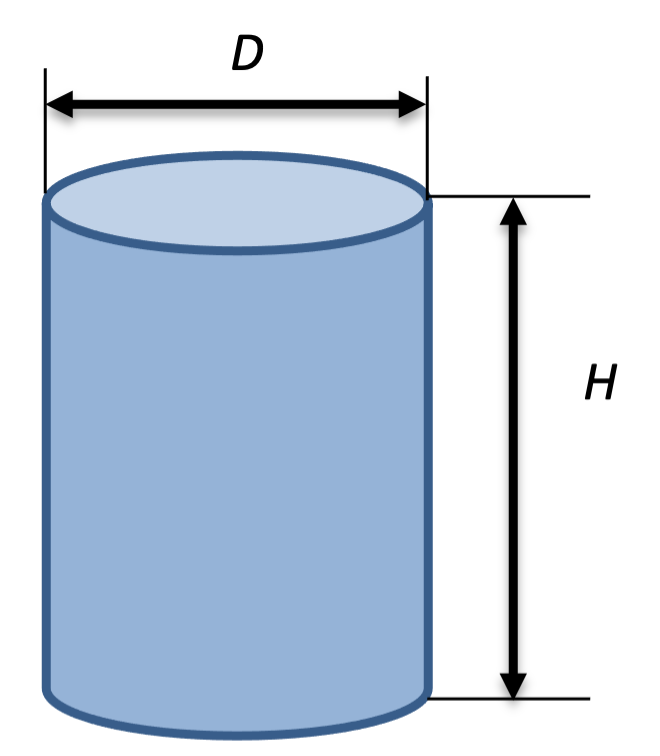
Surface area of the flat bottom part of the cylinder is calculated using Equation 2.1:

[latex]\begin{equation} A_{bottom} = \frac {\pi D^2}{4} \qquad \qquad \qquad \qquad (Eq. \thinspace 2.1) \end{equation}[/latex]

Then, force exerted by this object on the flat surface is calculated as shown in Equation 2.2:

[latex]\begin{equation} F_{g} \thinspace = mg \qquad \qquad \qquad \qquad \left( Eq.2.2 \right) \end{equation}[/latex]

where F_g is the force of gravity or weight of the object in units of Newton (N), m is the mass in units of kilograms (kg), and g = 9.81 m/s² is the acceleration due to gravity.

Finally, pressure is calculated using Equation 2.3:

[latex]\begin{equation} P = \frac {F_g}{A_{bottom}} = \frac {F_g}{\pi D^2 / 4} = \frac {4F_g}{\pi D^2} \qquad \qquad \qquad (Eq. \thinspace 2.3) \end{equation}[/latex]

where P is the pressure in units of Pascal, F_g is the weight of the object in Newtons, and D is the diameter of the cylindrical object in meters.

For example, let’s calculate the pressure exerted by the cylindrical object with a diameter of 4.5 cm and mass of 54.0 g on a flat surface.

First, convert a measurement in units of grams (g) to kilograms (kg) as follows:

[latex]\begin{equation} 1 = \frac {0.001 kg}{1g} = \frac {1 kg}{1,000g} \end{equation}[/latex]

or, simply divide the mass in grams by 1,000 g/kg as indicated below:

[latex]\begin{equation} m = 54.0g = \frac {54.0g}{1,000g/kg} = 0.0540 kg = 5.40 \times 10^{-2} kg \end{equation}[/latex]

Note that the answer above is given in scientific notation with three significant digits.

Next, calculate the weight of the object as:

[latex]\begin{equation} F_{g} = (0.0540 kg) \times (9.81 m/s^2) = 0.529 N \end{equation}[/latex]

Before calculating the pressure, we need to convert diameter from centimeters to meters using the conversion factor 1 m = 100 cm or, simply, dividing the measurement in units of centimeters by 100 cm/m to obtain meters:

[latex]\begin{equation} D = 4.5 cm = \frac {4.5 \cancel{cm}}{100 \cancel{cm}/m} = 0.045 m = 4.5 \times 10^{-2} m \end{equation}[/latex]

Then calculate the area of the bottom part of the object that comes in contact with the flat surface using Equation 2.1:

[latex]\begin{equation} A_{bottom} = \frac {\pi \left( 0.045m \right)^2}{4} = 0.00159 m^2 = 1.59 \times 10^{-3} m^2 \end{equation}[/latex]

And, finally, the pressure exerted by the cylindrical object in contact with the flat surface is calculated using Equation 2.3 to two significant digits:

[latex]\begin{equation} P = \frac {0.529 N}{0.00159 m^2} = 330 Pascal = 3.30 \times 10^2 Pa \end{equation}[/latex]

When the object expands as the pressure surrounding it decreases under vacuum conditions, its weight does not change, but A_bottom will get larger which will result in less pressure on the flat surface.

Equipment and Materials

1. 1 Ruler
2. 1 Mass Scale or Mass Balance
3. 1 Marshmallow
4. RVET (rough vacuum equipment trainer)

Procedure

Learning Activity 2.1: Pressure Exerted by the Marshmallow on a Table Top

Learning Activity 2.2: Components of the Rough Vacuum Equipment Trainer System

Learning Activity 2.3: Gas Flow Paths

What states do the following components need to be in for the system to be venting? You may watch Video 2.3 to help answer this question.

a. Vacuum chamber:	open	closed	doesn’t matter
b. Roughing valve:	open	closed	doesn’t matter
c. Venting valve:	open	closed	doesn’t matter
d. Roughing pump:	open	closed	doesn’t matter